Question from Rachel:
Question involving absolute value in Algebra 2: 3.4.
Example 5 on page 233 states that “If X<0, then |x| = -x.
That really confused me. I thought that absolute value was just that – absolute! There are not supposed to be any negatives involved, right?
But obviously, there are, and I’m not sure why. I’d appreciate some help. Thank you!
Answer from Dr. Callahan:
I know it looks confusing – but note that they are NOT saying |x| = -x — but instead they are looking for a trick to help break up the complex function into pieces.
See top of page 232.
This is saying that anytime you have a function with absolute value – you know it is likely to be a piecewise function – in other words it will break somewhere like this one does
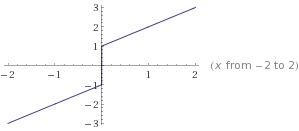To get NORMAL functions, we need to break this into two parts (sometimes more) so we can get an equation like on top of page 234 – a piecewise arrangement.
So this replacement is mostly a trick to make it work.
For instance – think about breaking up
y = |x| into piecewise.
It looks like this
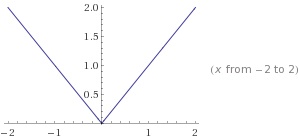To write the function, we have one part for x >= 0 and then one part for x<0.
To find this we let |x| = x when x>=0 and then |x| = -x when x<0. This swaps the signs for you – a trick. Try it by making a table of x and y for value positive an negative of y = |x|.
So again – this is more a trick to break it up than a FACT. See in the example right next the the word SOLUTION is says we are trying to break this up into a form that does NOT involve |x|.
